How to... (step-by-step instructions)
      Back to home
      1. Pix4D Documentation
      2. PIX4Dmatic
      3. How to... (step-by-step instructions)

      How are the Internal and External Camera Parameters defined? -PIX4Dmatic

      This article describes in detail the mathematical models behind the camera's internal and external parameters for PIX4Dmatic

      IN THIS ARTICLE

      Camera external parameters
      Camera internal parameters
           Perspective lens
             Camera without distortion model
             Camera with distortion model
           Fisheye lens

      Camera external parameters

      The external camera parameters are different for each image. They are given by:

      • T = (Tx, Ty, Tz) the position of the camera projection center in world coordinate system.
      • R the rotation matrix that defines the camera orientation with angles ω, φ, κ (PATB convention).

      If X = (X, Y, Z) is a 3D point in world coordinate system, its position X' = (X', Y', Z') in camera coordinate system is given by:

      Figure 1. 3D geometry of camera externals. Looking from T towards the 3D point X shows the image as seen on the screen. The world coordinate system is defined as Z pointing up, Y pointing North, and X pointing East.
       

      Camera internal parameters: Perspective lens

      Camera without distortion model

      The pixel coordinate (xu, yu) of the 3D point projection without distortion model is given by:

      Where f is the focal length in pixel, and (cx, cy) the principal point in pixel coordinates.

      Figure 2. Geometry of a perspective camera without distortion. Looking from T' towards the 3D point X' shows the image as seen on the screen, the origin of the image coordinate system is at the lower-left corner of the image. The image coordinates systems (X', Y', Z') in Figures 1 and 2 correspond.

      Camera with distortion model

      Let:

      be the homogeneous point,

      the squared 2D radius from the optical center, R1, R2, R3 the radial and T1, T2 the tangential
      distortion coefficients. The distorted homogeneous point in camera coordinate system (xhd, yhd)
      is given by:

      The pixel coordinate (xd, yd) of the 3D point projection with distortion model is given by:

      Where f is the focal length in pixel, and (cx, cy) the principal point in pixel coordinates.

      Fisheye lens

      The distortion for a fisheye lens is defined by:

      • The parameters C, D, E, F that describe an affine deformation of the circular image in
        pixel coordinates.
        The diagonal elements of the affine matrix can be related to the focal length f:


        The off-diagonal elements are connected to the distortion of the projected image circle,
        which, in the most general case, can be a rotated ellipse.

      • The coefficients p2, p3, p4 of a polynomial:

        Where:

      The pixel coordinate (xd, yd) of the 3D point projection with a fisheye distortion model is
      given by

      Where:

      And (cx, cy) is the principal point in pixel coordinates.

      Example:

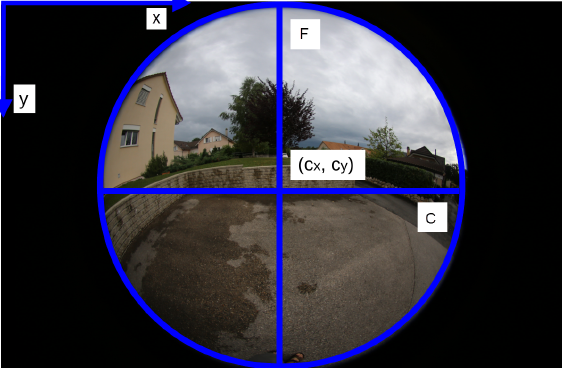
      When using an 8mm Sigma lens on a Canon 6D camera with an image size of 5472 x 3648 pixel(figure. 3), the internal parameters can be initialized as follows:

      • (cx, cy) = ( 5472/2, 3648/2 ) pixel is the center of the projected image circle
      • p2 = p3 = p4 = 0
      • p1 = 1
      • C = F = 1780 pixel is the radius of the image circle
      • E = D = 0
      Figure 3. The distortion of an 8mm Sigma lens on a Canon 6D.